

For typical commercial aircraft applications the Reynolds number per foot of chord length lies between 1.5 and 2 million per foot, as illustrated in Figure 5.21. Using the information on the atmosphere presented in Appendix B, one may determine the ratio V*/ν as a function of altitude and then show that Re c/ Mc = 7 × 10 6exp(− z/32,000), where z is the altitude in feet, represents a good fit to the atmospheric data.
However, it is important to recognize the assumptions which have been made, so that if a metering situation deviates from what has been assumed, a “flag will go up” to indicate that the effect of Reynolds number must be evaluated and treated. These equations can be combined and rewritten in simplified forms. These effects will be further discussed in the sections covering the description and application of different meters, in Chapters 8, 9, and 10 Chapter 8 Chapter 9 Chapter 10, and the equations will be covered more thoroughly later in this book. To completely define the meter’s application there must be no deformed profiles, such as after an elbow or where upstream piping has imparted swirl to the stream. Thus, calculation of the Reynolds number will define the flow velocity pattern and approximate limits of the meter’s application. At 4,000 and above the flow is in the turbulent flow area and the profiles are fairly flat. Between 2,000 and 4,000 the flow is in the transition region. At values of 2,000 and below, the flow profile is bullet-shaped (parabolic). Units in the pound, foot, second system are shown below:īased on Reynolds’ work, the flow profile (which affects all velocity-sensitive meters and some linear meters) has several important values. Note: All parameters are given in the same units, so that when multiplied together they all cancel out, and the Reynolds number has no units. Rather it has to stay with the streamline and will be dragged along.Īs the Reynolds number is so important for microfluidics, we will detail its meaning and application when discussing the concept of dimensional analysis in section 11.8.3. A good example is a large vessel or ship (with Reynolds numbers in the range of 1 × 10 9) compared to a folded origami or paper boat: the large vessel can force its way through the current and the waves, whereas the light paper boat would not be able to do so. At higher Reynolds numbers, an object is able to force its way through a flow field even across the streamlines. For most fish, the Reynolds number is in the range of 1 × 10 5, for a human it is in the range of 1 × 10 6.
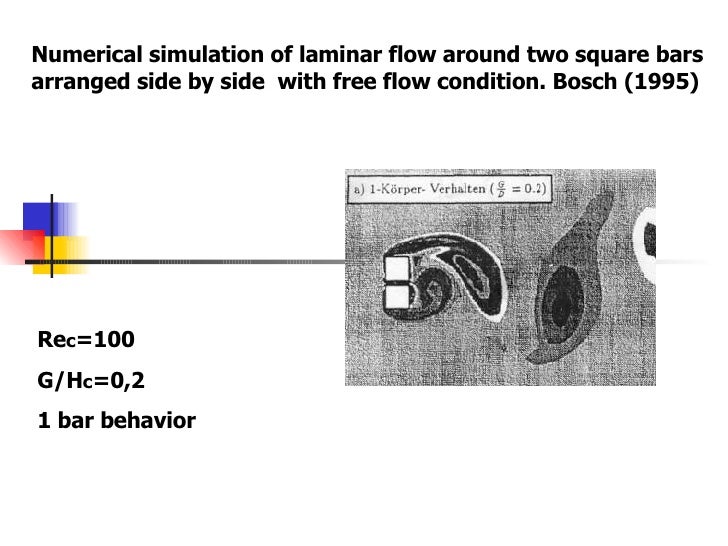
As the objects grow larger, their inertia starts to dominate over the viscous forces. For such objects, a fluid would feel significantly more rigid, i.e., it would be difficult for a bacteria to force a path through a moving fluid not following the streamlines. Given the small dimensions, these objects do not have a significant inertia and are thus mainly driven by the viscous forces of the fluid. As an example, for very small organism, e.g., bacteria, the Reynolds number is very small, typically in the range of 1 × 10 −6. The Reynolds number is important for describing the transport properties of a fluid or a particle moving in a fluid. 9.20) Re = ρ υ L char η = υ L char v = inertia forces viscous forces = Pe Se


 0 kommentar(er)
0 kommentar(er)
